The letters FAVER are an acronym for Fixed Axis Vertically Rotated. This is the 4th type of random section in use in stereology today. Unlike a VUR section, in a FAVER section the vertical axis is fixed. The orientation of the vertical axis in a VUR section is fixed, but the vertical axis about which the sample was rotated does not necessarily appear in the VUR section. It does in a FAVER section.
FAVER sections are created using the following method:
- Elect a vertical axis and a point through which the vertical axis passes. This fixes the position and the orientation of the axis. Typically, the axis and point are chosen based on the geometry of the material being studied. A piece of bone has a clear central axis. Notice that the choice of the axis is arbitrary and no randomness if involved.
- Rotate the object about the axis. The rotation should be random. This is where the method is randomized.
- Take a thick slice about the axis or take two consecutive sections. Consecutive sections are adjacent sections.

The values associated with the FAVER section are shown here. This is a top view. The axis is the dot int h center of the circle. The thickness of the slice or the distance between adjacent sections is shown as h. The distance from the axis to a particle is d. Particles at a distance d lie on a circle. Only a portion of the circle lies within the FAVER section. The parts of the circle that lie within the FAVER section can be approximated by h, since the arc is nearly a straight line.

Be aware that d is being used for distance and not diameter. The distance is the radius of the circle. The approximation that the arc length is h is reasonable for most particles.
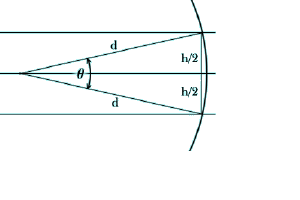
This is a drawing of the FAVER section showing the circle arc and the use of h as the length of the arc. The length of the arc is equal to the radius of the circle times the angle theta. The angle theta can be computed by taking the arc-sine of the ratio of the far side of the triangle to the hypotenuse.
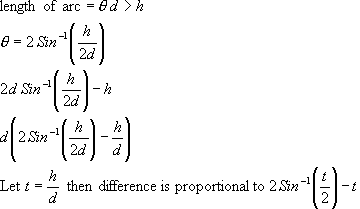
A substitution was made for the ratio of h, the thickness, to d, the distance from the axis. A plot of that final equation shows that the error is less than 0.001 when the particle is 4 times the thickness from the axis. The error continues to drop to near 0 just 20 times the thickness. For example, suppose that the FAVER section is 50 microns. By 200 microns from the axis, the error is extremely small. By 1000 microns, the error is nonexistent for all practical purposes.
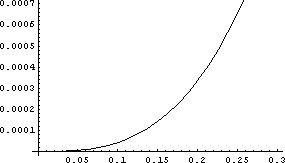
Knowing the probability of a particle being selected makes it possible to estimate the number of particles in a population. The Thompson estimator can be applied here. A particle has a weight of 1. The probability is given above.
____________________________________________________________________

Sponsored by MBF Bioscience
developers of Stereo Investigator, the world’s most cited stereology system